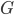(This is a work in progess…) Nth roots of Unity \(n^{th}\) roots of unity is given by the equation:\(\large (1)^{1\over n}=e^{i\frac{2k\pi}{n}}\), \(\large k=0,1,\dots,n-1\) Applet for visualizing nth roots of unity Nth Cyclotomic Polynomial \(n^{th}\) cyclotomic polynomial, for any positive integer \(n\), is the unique irreducible polynomial with integer coefficients that is a divisor of \(x^{n}-1\) and is not a divisor of \(x^{k}-1\) for any \(k < n\). Its roots are…
Category: Algebra
Useful Propositions of Group Theory

(This is a work in progess…) Rearrangement Theorem: \(R\bf{G} = \bf{G}\) \((\forall R \in \bf{G})\). Equivalent statement about the columns being \(\bf{G} R = \bf{G}\) \((\forall R \in \bf{G})\) Each row and each column in the group multiplication table lists each of the group elements once and only once. From this, it follows that no two elements may be in the identical location in two rows or two columns. Thus,…
Is abelian group \(G\) always isomorphic to H×(G/H)?

Let \(G= \mathbb{Z}_4=\mathbb{Z}/4\mathbb{Z}\) and \(H=\langle2\rangle\) Then \(G= \mathbb{Z}_4 = \mathbb{Z}/4 \mathbb{Z} = \{0,1,2,3\}\).We know \(\mathbb{Z}/4\mathbb{Z}\) is cyclic. Cayley Table of \(G=\mathbb{Z}/4 \mathbb{Z}\) is:\(\begin{array}{|c|c|c|c|c|}\hline\hline\textbf{+} & \textbf{0} & \textbf{1} & \textbf{2} & \textbf{3}\\\hline \textbf{0} & 0 & 1 & 2 & 3 \\\hline \textbf{1} & 1 & 2 & 3 & 0 \\\hline \textbf{2} & 2& 3 & 0 & 1\\\hline\textbf{3} & 3 & 0 & 1 & 2 \\\hline \end{array}\) \(\cong\) \(\begin{array}{|c|c|c|c|c|}\hline\hline\bf{+}…
Some notes on Polynomials

(This is a work in progess…) (Note: I am doing some exploration on Galois Theory and I have written these notes on polynomials here for my own convenience. Please write in the comments section if any clarification is required. Also, I will not provide proofs here for the time being.) Some notations: The symbol \(\mathbb{C}\) is the domain of complex numbers; i.e. set of all possible complex numbers.The symbol \(\mathbb{R}\) is…
Group homomorphism and examples

Group A group is any set G with a defined binary operation (called the group law of \(G\)), written as 2 tuple (examples: \((G,*), (G,\cdot), (G,+), …\)), satisfying 4 basic rules Closure The important point to be understood about a binary operation on \(G\) is that \(G\) is closed with respect to \(*\) in the sense that if \(a,b\in G\) then \(a*b\in G\) (\(a,b\in{C}\) can be read as “a,b element…
A Day out with FreePlane Mindmap and Mathematical Groups

Background I wanted to break away from Blender Learning/Modeling (Current passion) for a day or two. And so I began my exploration in Mathematical Groups using FreePlane Mind mapping software. I had recently switched from Freemind to to Freeplane. There was a simple enough reason for this decision – LaTeX support. Freeplane allows us to enter Mathematical equations using LaTeX. But a certain disadvantage of using Freeplane is that you…