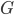Let \(G= \mathbb{Z}_4=\mathbb{Z}/4\mathbb{Z}\) and \(H=\langle2\rangle\)
Then \(G= \mathbb{Z}_4 = \mathbb{Z}/4 \mathbb{Z} = \{0,1,2,3\}\).
We know \(\mathbb{Z}/4\mathbb{Z}\) is cyclic.
Cayley Table of \(G=\mathbb{Z}/4 \mathbb{Z}\) is:
\(\begin{array}{|c|c|c|c|c|}\hline\hline\textbf{+} & \textbf{0} & \textbf{1} & \textbf{2} & \textbf{3}\\\hline \textbf{0} & 0 & 1 & 2 & 3 \\\hline \textbf{1} & 1 & 2 & 3 & 0 \\\hline \textbf{2} & 2& 3 & 0 & 1\\\hline\textbf{3} & 3 & 0 & 1 & 2 \\\hline \end{array}\) \(\cong\) \(\begin{array}{|c|c|c|c|c|}\hline\hline\bf{+} & \bf{I} & \bf{V_1} & \bf{V_2} & \bf{V_3}\\\hline \bf{I} & I & V_1 & V_2 & V_3 \\\hline \bf{V_1} & V_1 & V_2 & V_3 & I \\\hline \bf{V_2} & V_2& V_3 & I & V_1\\\hline\bf{V_3} & V_3 & I & V_1 & V_2 \\\hline \end{array}\)
And \(H=\langle2\rangle=\{0,2\}\).
Cayley Table of \(H=\{0,2\}\) is:
\(\begin{array}{|c|c|c|c|c|} \hline \hline \bf{+} &\bf{0}&\bf{2}\\ \hline \bf{0}&0&2\\\hline \bf{2} &2&0\\ \hline \end{array}\)
Then, \(G/H=\{\{0,2\},\{1,3\}\}\).
So, the group structures of \(H, G/H, \mathbb{Z}_2\) are Isomorphic.
That is \(H\cong G/H\cong\mathbb{Z}_2\).
And \(H\times G/H=\{(0,\{0,2\}),(2,\{0,2\}),(0,\{1,3\}),(2,\{1,3\})\}\).
\((0,\{0,2\})\) is Identity in \(H\times G/H\).
Cayley Table of \(H\times G/H\) is:
\(\begin{array}{|c|c|c|c|c|} \hline \hline \bf{+} &\bf{(0,\{0,2\})}&\bf{(2,\{0,2\})}&\bf{(0,\{1,3\})}&\bf{(2,\{1,3\})}\\ \hline \bf{(0,\{0,2\})} &(0,\{0,2\})&(2,\{0,2\})&(0,\{1,3\})&(2,\{1,3\})\\ \hline \bf{(2,\{0,2\})} &(2,\{0,2\})&(0,\{0,2\})&(2,\{1,3\})&(0,\{1,3\})\\ \hline \bf{(0,\{1,3\})} &(0,\{1,3\})&(2,\{1,3\})&(0,\{0,2\})&(2,\{0,2\})\\ \hline \bf{(2,\{1,3\})} &(2,\{1,3\})&(0,\{1,3\})&(2,\{0,2\})&(0,\{0,2\})\\ \hline \end{array}\)
The Group structure of \(H\times G/H\) is Isomorphic to \(\mathbb{Z}_2\times\mathbb{Z}_2\):
\(\begin{array}{|c|c|c|c|c|} \hline \hline \bf{+} & \bf{I} & \bf{V_1} & \bf{V_2} & \bf{V_3} \\ \hline \bf{I} & I & V_1 & V_2 & V_3 \\ \hline \bf{V_1} & V_1 & I & V_3 & V_2 \\ \hline \bf{V_2} & V_2 & V_3 & I & V_1 \\ \hline \bf{V_3} & V_3 & V_2 & V_1 & I \\ \hline \end{array}\)
Therefore, \(H\times G/H \cong \mathbb{Z}_2\times\mathbb{Z}_2\) is not isomorphic to \(\mathbb{Z}_4=\mathbb{Z}/4\mathbb{Z}\).