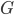(This is a work in progess…) Nth roots of Unity \(n^{th}\) roots of unity is given by the equation:\(\large (1)^{1\over n}=e^{i\frac{2k\pi}{n}}\), \(\large k=0,1,\dots,n-1\) Applet for visualizing nth roots of unity Nth Cyclotomic Polynomial \(n^{th}\) cyclotomic polynomial, for any positive integer \(n\), is the unique irreducible polynomial with integer coefficients that is a divisor of \(x^{n}-1\) and is not a divisor of \(x^{k}-1\) for any \(k < n\). Its roots are…
Category: Group Theory
Useful Propositions of Group Theory

(This is a work in progess…) Rearrangement Theorem: \(R\bf{G} = \bf{G}\) \((\forall R \in \bf{G})\). Equivalent statement about the columns being \(\bf{G} R = \bf{G}\) \((\forall R \in \bf{G})\) Each row and each column in the group multiplication table lists each of the group elements once and only once. From this, it follows that no two elements may be in the identical location in two rows or two columns. Thus,…
Is abelian group \(G\) always isomorphic to H×(G/H)?

Let \(G= \mathbb{Z}_4=\mathbb{Z}/4\mathbb{Z}\) and \(H=\langle2\rangle\) Then \(G= \mathbb{Z}_4 = \mathbb{Z}/4 \mathbb{Z} = \{0,1,2,3\}\).We know \(\mathbb{Z}/4\mathbb{Z}\) is cyclic. Cayley Table of \(G=\mathbb{Z}/4 \mathbb{Z}\) is:\(\begin{array}{|c|c|c|c|c|}\hline\hline\textbf{+} & \textbf{0} & \textbf{1} & \textbf{2} & \textbf{3}\\\hline \textbf{0} & 0 & 1 & 2 & 3 \\\hline \textbf{1} & 1 & 2 & 3 & 0 \\\hline \textbf{2} & 2& 3 & 0 & 1\\\hline\textbf{3} & 3 & 0 & 1 & 2 \\\hline \end{array}\) \(\cong\) \(\begin{array}{|c|c|c|c|c|}\hline\hline\bf{+}…
Group homomorphism and examples

Group A group is any set G with a defined binary operation (called the group law of \(G\)), written as 2 tuple (examples: \((G,*), (G,\cdot), (G,+), …\)), satisfying 4 basic rules Closure The important point to be understood about a binary operation on \(G\) is that \(G\) is closed with respect to \(*\) in the sense that if \(a,b\in G\) then \(a*b\in G\) (\(a,b\in{C}\) can be read as “a,b element…